Abstract

This study examines the benefits of adding microencapsulated phase change material (PCM) to concrete used in building envelopes to reduce energy consumption and costs. First, it establishes that the time-dependent thermal behavior of microencapsulated PCM-concrete composite walls can be accurately predicted by an equivalent homogeneous wall with appropriate effective thermal properties. The results demonstrated that adding microencapsulated PCM to concrete resulted in a reduction and a time-shift in the maximum heat flux through the composite wall subjected to diurnal sinusoidal outdoor temperature and solar radiation heat flux. The effects of the PCM volume fraction, latent heat of fusion, phase change temperature and temperature window, and outdoor temperature were evaluated. Several design rules were established including (i) increasing the PCM volume fraction and/or enthalpy of phase change increased the energy flux reduction and the time delay, (ii) the energy flux reduction were maximized when the PCM phase change temperature was close to the indoor temperature, (iii) the optimum phase change temperature to maximize the time delay increased with increasing average outdoor temperature, (iv) in extremely hot or cold climates, the thermal load could be delayed even though energy flux reduction was small, and (v) the choice of phase change temperature window had little effect on the energy flux reduction and the time delay. This analysis can serve as a framework to design PCM composite walls in various climates and seasons and to take advantage of time of use electricity pricing.

Effective heat capacity method for simulating phase change

To account for the latent heat stored during phase transition, the PCM specific heat can be defined as a piecewise function of temperature given by the effective heat capacity method and shown in Figure 1. This is an idealized representation of the PCM specific heat. In reality, PCMs exhibit superheating and subcooling during melting and freezing, respectively, delaying phase change and causing hysteresis in the c_p,c(T) plot.

Schematics and Assumptions

Figure 2 illustrates a heterogeneous slab of a three-component composite material consisting of aligned unit cells of monodisperse microcapsules filled with PCM and embedded in a concrete matrix in an FCC packing arrangement and an equivalent homogeneous slab with some effective thermal properties. It also shows the associated coordinate system and the boundary conditions used to validate the effective homogeneous model. The overall thickness of this composite slab was denoted by L.

Figure 2. Schematic of a heterogeneous slab consisting of aligned unit cells of microencapsulated PCM distributed in concrete with a face-centered cubic (FCC) packing arrangement and an equivalent homogeneous slab with some effective thermal conductivity k_eff and effective volumetric heat capacity (ρc_p)_eff..

To make the problem mathematically tractable, the following assumptions were made: (1) All materials were isotropic and had constant properties except for the temperature-dependent PCM specific heat. (2) The PCM specific heat was the same for solid and liquid phases. (3) Interfacial contact resistances between the concrete, the shell, and the PCM were negligible. (4) Natural convection in the molten microencapsulated PCM was absent based on the fact that the Rayleigh number was very small. (5) There was no heat generation in the wall.

Governing equations and boundary conditions

The temperature T in the homogeneous material equivalent to the heterogeneous wall of identical dimensions was governed by

where α_eff(T) = k_eff / (ρc_p)_eff(T) and is the effective thermal diffusivity. Note that α_eff(T) is a function of the local temperature T since it accounts for the temperature-dependent specific heat of the PCM. However, a consistent model for the effective volumetric heat capacity (ρc_p)_eff and the effective thermal conductivity k_eff of the composite wall needs to be specified. To approximate the heterogeneous composite by a homogeneous medium with some effective thermal properties, its effective volumetric heat capacity can be expressed as,

where ϕ_c and ϕ_s are the volume fractions of the core and shell materials, while (ρc_p)_c, (ρc_p)_s, and (ρc_p)_m are the volumetric heat capacities of the core, shell, and matrix materials, respectively. By defining c_p,c(T) based on the effective heat capacity method, (ρc_p)_eff(T) can be expressed as,

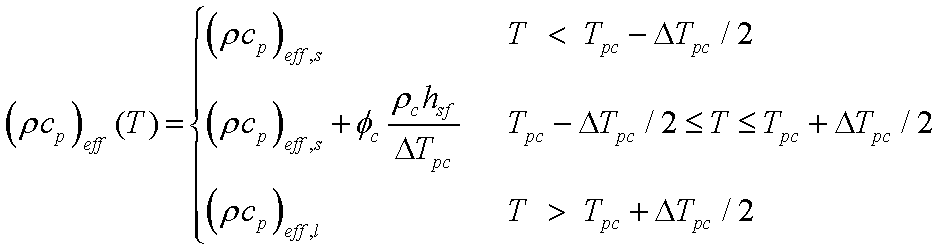
where (ρc_p)_eff,s is the effective volumetric heat capacity of the PCM-concrete composite wall with unmelted PCM given by

Here, it was assumed to be equal to the effective volumetric heat capacity of the composite with fully melted PCM, i.e., (ρc_p)_eff,s = (ρc_p)_eff,l. Moreover, Felske [1] used the self-consistent field approximation to derive an effective medium approximation (EMA) to predict the effective thermal conductivity k_eff of monodisperse spherical capsules randomly distributed in a continuous matrix given by,

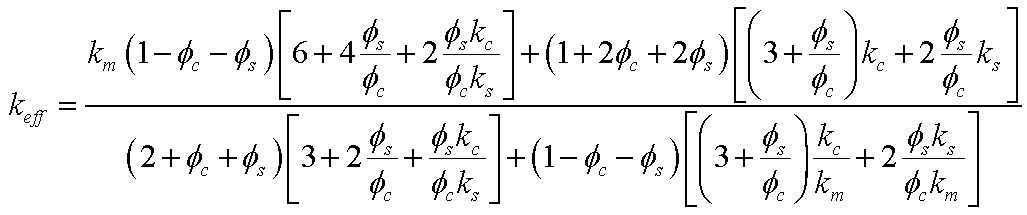
This expression was validated using detailed numerical simulations of ordered and randomly distributed monodisperse and polydisperse microcapsules. Convective heat transfer was imposed at the interior wall surface x = L with a constant indoor temperature T_in maintained by the HVAC system so that,

where h_i is the indoor mixed convective heat transfer coefficient accounting for both forced and natural convections. Combined convective and radiative heat transfer was imposed at the exterior wall surface given by,

where h_o is the outdoor convective heat transfer coefficient, T_sky represents the average sky temperature, α_s and ε are the total hemispherical absorptivity and emissivity of the outdoor wall surface, respectively, and σ is the Stefan-Boltzmann constant, i.e., σ = 5.67x10^-8 W/m²K⁴. The ambient outdoor temperature was imposed as a sinusoidal function of time t (in s) expressed as,

where T_max and T_min are the maximum and minimum outdoor temperatures during a day, respectively. A phase shift of 2π/3 placed the peak outdoor temperature T_max at 2:00 pm, as the daily maximum occurred between 1:00 pm and 3:00 pm for more than 80% of the year in California climate zone 9 (Los Angeles, CA) based on weather data. Similarly, the solar radiation heat flux q"_s(t) as a function of time t (in s) was imposed as,

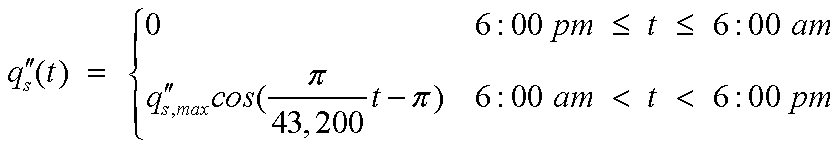
where q"_s,max(t) is the maximum daily solar radiation heat flux (in W/m²). Here, the maximum daily solar radiation heat flux q"_s,max(t) was taken as 535 W/m² and occurred at 12:00 pm corresponding to the average daily maximum value and time throughout the year in California climate zone 9.

Results and Discussion

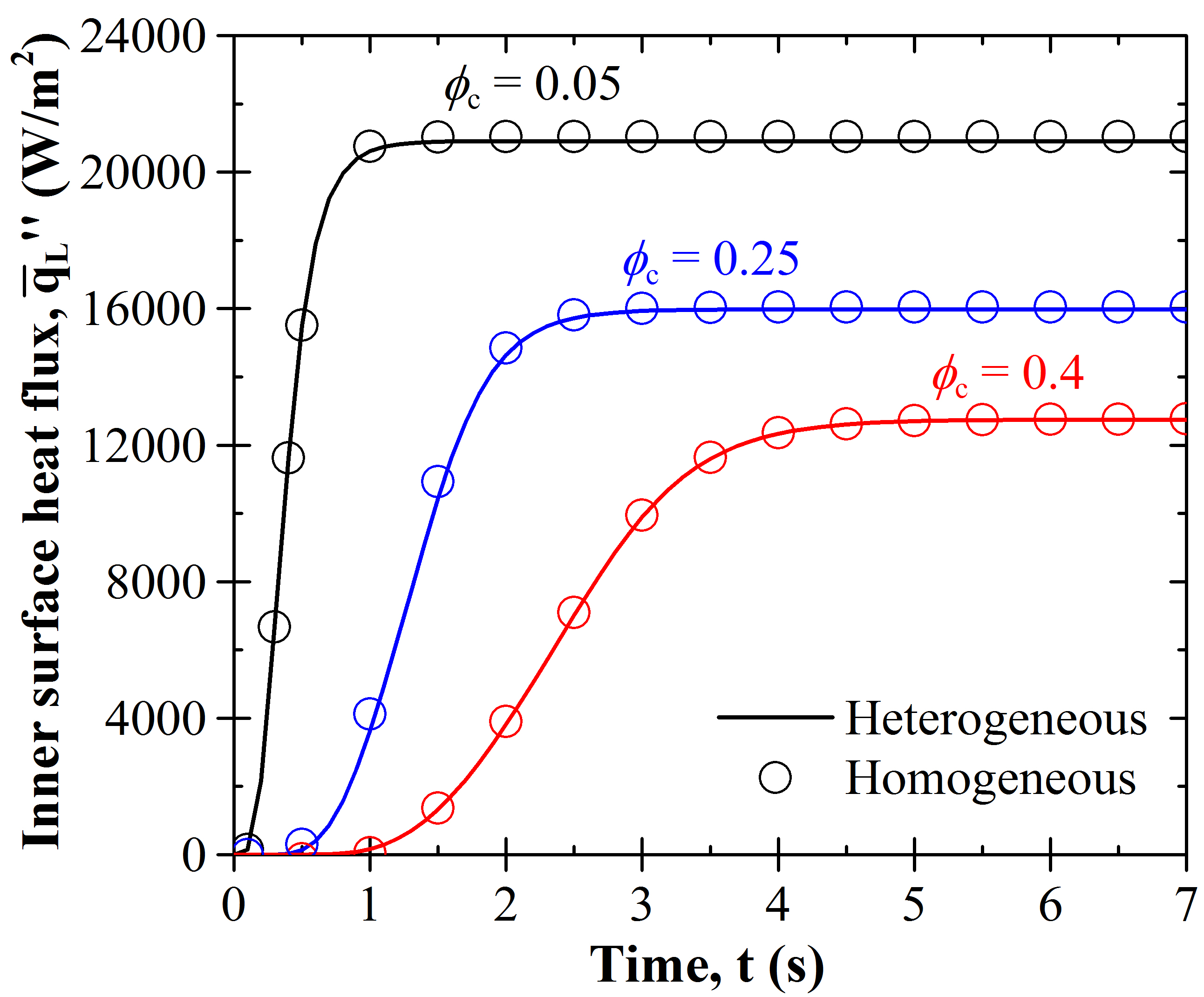
Figure 3 shows the area-averaged inner surface heat flux as a function of time for PCM volume fraction ϕ_c of 0.5, 0.25, and 0.4. It also shows the corresponding predictions of the heat flux for the equivalent homogeneous slab with the above mentioned effective thermal properties. In all cases, the average relative difference in the predicted area-averaged inner surface heat flux between the heterogeneous composite slab and the equivalent homogeneous slab was less than 2% at all times when the inner surface heat flux was greater than 5% of its steady-state value. Figure 3 also demonstrates that increasing the PCM volume fraction decreased the inner surface heat flux at all times and delayed its steady state. This can be attributed to the fact that adding PCM not only enhanced the latent and sensible thermal mass of the composite material but also its thermal resistance.

Figure 4a plots the inner wall heat flux as a function of time for PCM volume fraction ϕ_c ranging from 0.0 to 0.5. It demonstrates that increasing the PCM volume fraction significantly reduced the heat transfer through the wall. In fact, a PCM volume fraction ϕ_c of 0.5 reduced the range of variation in the inner wall heat flux by more than 90% compared with plain concrete. Figure 4b plots the energy flux reduction E_r as a function of PCM volume fraction ϕ_c ranging from 0.0 to 0.5. In the absence of phase change, E_r increased linearly with increasing ϕ_c due to the associated increase in thermal resistance and sensible heat storage of the wall, i.e., k_eff < k_m and (ρc_p)_eff,s > (ρc_p)_m. The energy flux reduction E_r was notably larger when phase change was accounted for. Figure 4c shows the time delay τ_d in the maximum inner wall heat flux as a function of PCM volume fraction ϕ_c. It indicates that τ_d increased significantly with increasing PCM volume fraction, reaching more than 13 hours for ϕ_c of 0.5. Here also, the contribution of phase change to the time delay was important and dominated over that of other thermal effects.

Figure 4. (a) Inner wall heat flux q"_L(t) as a function of time through a 10 cm thick microencapsulated PCM-concrete wall subjected to sinusoidal diurnal boundary conditions with T_min=10^°C and T_max= 30^°C. (b) Energy flux reduction E_r and (c) time delay τ_d for PCM volume fraction ϕ_c ranging from 0.0 to 0.5. Here, h_sf = 180 kJ/kg, T_pc = 20^°C, and ΔT _pc=3^°C. The PCM specific heat was either constant and equal to (ρc_p)_eff,s or temperature-dependent to assess the effects of phase change.

Figure 5a plots the energy flux reduction E_r as a function of phase change temperature T_pc for average outdoor temperature (T_max + T_min)/2 of 10, 20, and 30^°C and for ϕ_c=0.1, h_sf = 180 kJ/kg, and ΔT_pc = 3^°C. For an average outdoor temperature of 20^°C, a distinct maximum energy flux reduction E_r,max of 39% was achieved for a phase change temperature T_pc equal to the indoor temperature T_in of 20^°C. Overall, there were no optimization opportunities in terms of energy reduction for a homogeneous PCM composite wall subjected to sinusoidal outdoor temperature oscillations and solar radiation heat flux in extremely hot or cold climates. Figure 5b plots the time delay τ_d as a function of phase change temperature T_pc for ϕ_c=0.1, h_sf = 180 kJ/kg, and ΔT_pc = 3^°C. In all cases, τ_d reached a maximum ranging from 2 to 3 hours as the average outdoor temperature decreased from 30 to 10^°C. Indeed, adding PCM to a building wall in an extremely hot or cold climate may still provide financial savings to the ratepayer if TOU pricing is available, by shifting the heating or cooling load to an off-peak time of day.

Figure 5. (a) Energy flux reduction E_r and (b) time delay τ_d as a function of phase change temperature T_pc for average outdoor temperature (T_max + T_min)/2 of 10, 20, and 30^°C and outdoor temperature amplitude (T_max - T_min)/2 of 10^°C. Here, ϕ_c = 0.1, h_sf = 180 kJ/kg, and ΔT_pc = 3^°C.

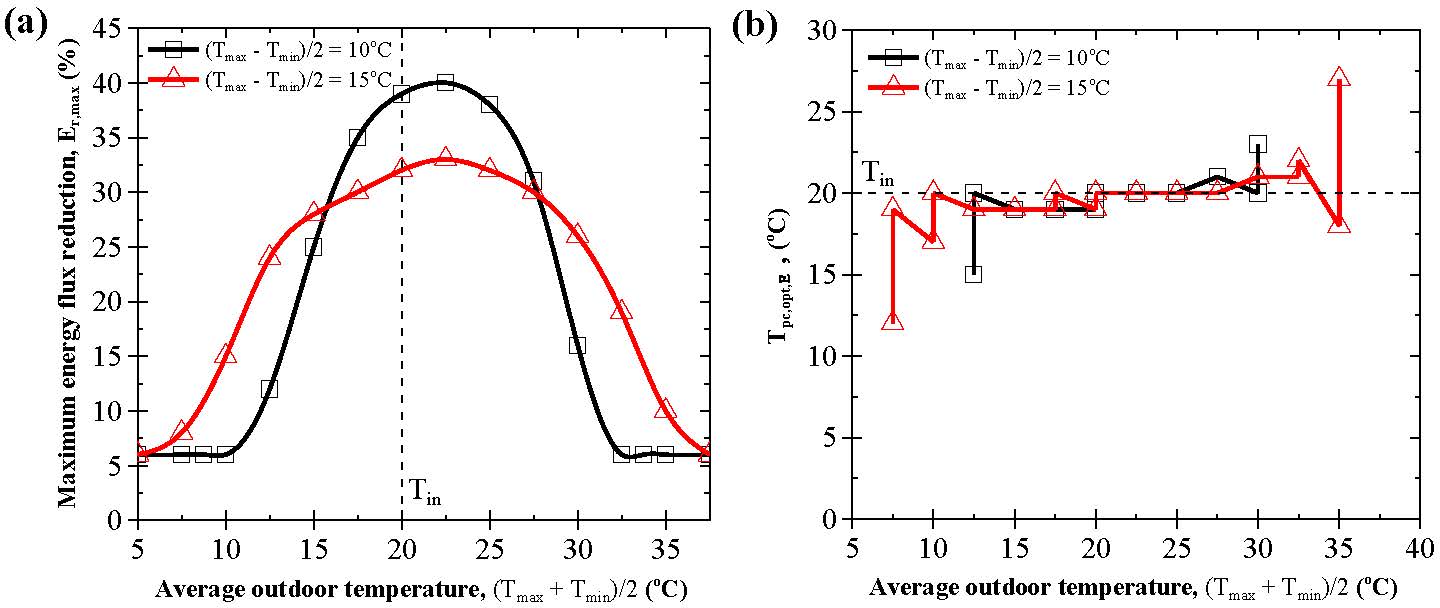
Figure 6: (a) Maximum energy flux reduction E_r,max and (b) phase change temperature corresponding to the maximum energy flux reduction T_pc,opt,Er for average outdoor temperatures ranging from 5 to 37.5^°C with an amplitude of either 10 or 15^°C. Here, ϕ_c = 0.1, h_sf = 180 kJ/kg, and ΔT_pc = 3^°C.

Conclusion

This study demonstrated that a composite wall containing microencapsulated PCMs can be accurately represented as a homogeneous wall with effective volumetric heat capacity (ρc_p)_eff thermal conductivity k_eff. This time-dependent homogeneous thermal model was used to establish important design rules that can inform the selection of microencapsulated PCMs for concrete walls in various climates. First, adding microencapsulated PCM to concrete walls and increasing the latent heat of fusion both substantially reduced and delayed the thermal load on the building. Second, the phase change temperature leading to the maximum energy flux reduction was equal to the desired indoor temperature regardless of the climate conditions. Third, to achieve the maximum time delay, the optimum phase change temperature increased with increasing average outdoor temperature. Fourth, in extremely hot or cold climates, the use of PCM delayed the thermal load to take advantage of TOU pricing even though the energy flux reduction were not significant. Lastly, the phase change temperature window had little effect on the energy flux reduction and the time delay. This analysis can inform future simulations of composite walls containing microencapsulated PCMs in any climate.

References

1. J.D. Felske, 2004. Effective thermal conductivity of composite spheres in a continuous medium with contact resistance. International Journal of Heat and Mass Transfer, Vol. 47, pp. 3453-3461. doi:10.1016/j.ijheatmasstransfer.2004.01.013
2. A.M. Thiele, A. Kumar, G. Sant, and L. Pilon, 2014. Effective Thermal Conductivity of Three-Phase Composites Containing Spherical Capsules. International Journal of Heat and Mass Transfer, Vol. 73, pp. 177-185. doi: 10.1016/j.ijheatmasstransfer.2014.02.002
3. A.M. Thiele, G. Sant, and L. Pilon, 2014. Diurnal Thermal Analysis of Microencapsulated PCM-Concrete Composite Walls. Energy Conversion and Management, Vol. 93, pp.215-227. doi:10.1016/j.enconman.2014.12.078